What is Wave Motion? 

A wave motion is defined as the propagation of disturbances or transfer of energy and momentum from the state of rest or equilibrium to another point in a medium without any actual transfer of matter between them. In a simple explanation, it is the propagation of energy through a medium that is produced due to the repeated vibrations of the particles. Common things we see day to day like waves on water oscillation of a pendulum or bell, light wave, and all the subatomic particles exhibiting wavelike properties. The propagation of waves depends on the medium and their properties. The wave function is the disturbance that produces waves as a function of time. This disturbance oscillates periodically with a fixed frequency and wavelength. There are linear wave motion and non-linear wave motion based on their propagation, motion, and time period where linear wave motion is like sinusoidal waves and non-linear wave motion like a wave motion that gets interrupted in the middle of their propagation. 

Types of Waves 

Wave motion is categorized on the basis of mode of propagation, the number of dimensions, characteristic of energy and momentum and based on the time period/periodicity. 

1) On the basis of mode of propagation  

They are classified into Mechanical waves and non-mechanical waves. Mechanical waves need a medium for its propagation and non-mechanical wave needs no medium, they can propagate in a vacuum too. They are also known as Elastic waves. These waves are transverse or longitudinal in nature. 

Mechanical wave are categorized into 4 types which are transverse waves, longitudinal waves, standing waves, and progressive waves. 

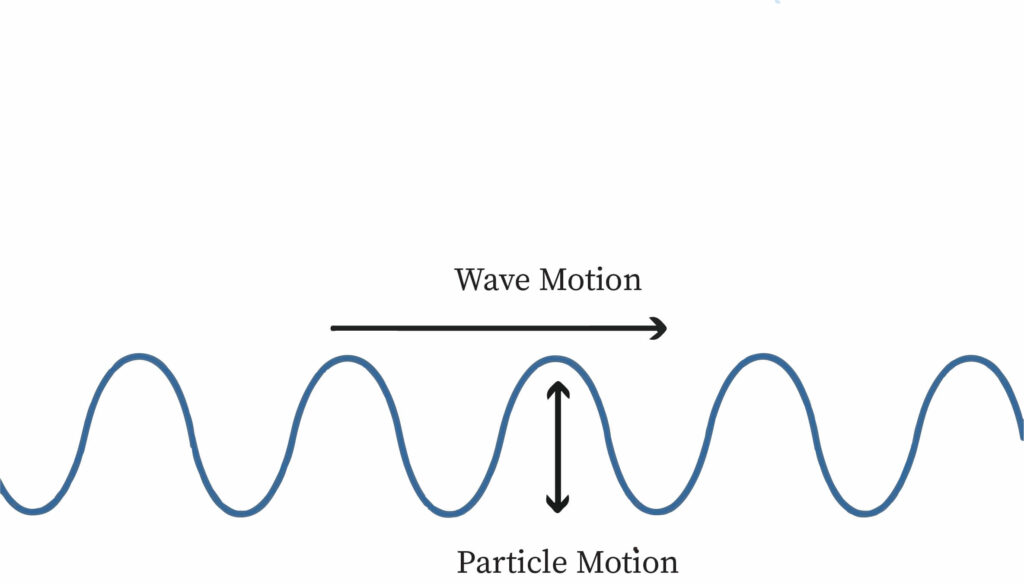
i) Transverse waves: It is a type of wave motion where all the particles in the wave oscillate or vibrate perpendicular to the direction of propagation of waves. The oscillation is always at a right angle to the direction. Example: Electromagnetic waves, surface ripples on the water 

ii) Longitudinal wave: It is a type of wave motion where all the particles in the wave oscillate or vibrate in the direction of propagation of waves. The displacement of the medium is the same as the direction of the wave propagating. Example: sound waves, seismic waves. 

2) On the basis of the characteristic of the transfer of energy 

i) Stationary wave: It is a type of wave motion which while exhibiting the disturbance doesn't transfer energy and momentum during its propagation from one medium to another medium. Example: The waves produced from musical instruments like guitar and violin 

ii) Progressive wave: It is a type of wave motion which while exhibiting the disturbance transfers its energy and momentum during its propagation from one medium to another medium. 

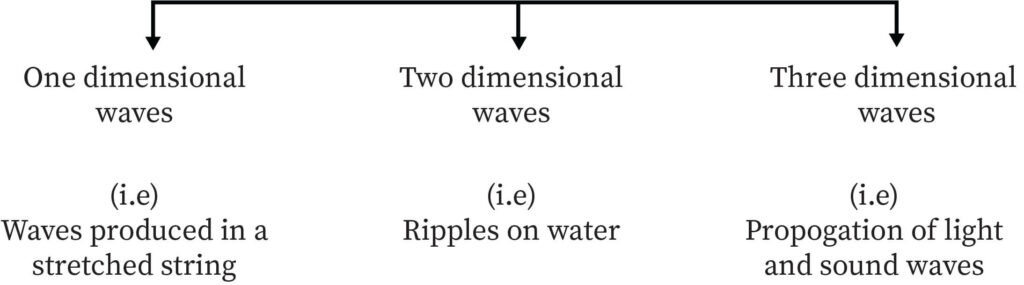
3) On the basis of the number of dimensions 

i) One-dimensional wave: A wave that oscillates or causes the disturbance to propagate only in one direction. Example: Plane-wave, like Oscillation of pendulum. 

ii) Two-dimensional wave: A wave that oscillates or causes the disturbance to propagate in a two-dimensional plane, in two directions x and y. Example: Circular wave, water waves 

iii) Three-dimensional wave: A wave that oscillates or causes the disturbance to propagate in a three-dimensional plane, in the directions x, y, and z. Example: Spherical waves like sound and light waves. 

4) On the basis of the time period 

 It is classified into two types: Periodic wave and non-periodic wave: 

i) A periodic wave is when the vibration caused is continuous, that is when the vibration of the wave repeats itself at regular intervals during its propagation, it usually behaves like a sinusoidal wave. The time interval of each vibration of the particle is the same. This repetitive movement to and fro motion from its equilibrium position like an oscillation of a simple pendulum is known as simple harmonic motion.  

ii) A non-periodic wave is when the vibration caused is not continuous, that is when the vibration of the wave does not repeat at regular intervals during its propagation. A very known example is speech signal. 

Wave Equation and their Characteristics 

• A wave equation is represented as the function y=asin(ωt-Φ)=a sin2π/λ(ct-x) or simply it can also be denoted as  y=asin(ωt-Φ) where a is the amplitude of the wave, ωt is the oscillation frequency and Φ is the phase angle of the wave. 
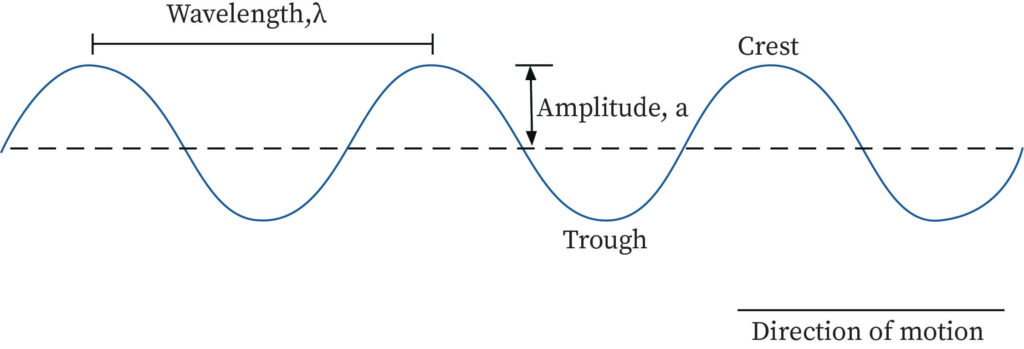
• The maximum displacement of the particle that is the disturbance caused from its equilibrium position is the amplitude of the wave motion. 
• The frequency of the wave is defined as the number of vibrations occurring per second by any particle in the medium which determines the character of the source producing the wave. The frequency remains constant throughout the propagation. 
• The time period t of the wave is the time taken by the particles to complete one cycle of oscillation of vibration. 
• The wavelength λ used in the wave equation is the distance between the particles in the medium which are in the same level of vibration that is the distance between two similar points in successive waves. 
• The phase angle φ is defined as the state of vibration of each and every particle in the medium vibrating or oscillating about its  mean position  
• The path difference of a wave is denoted as Δ x stating the difference between the positions of two particles measured of the wave traveling through the medium, Δ x=(x2-x1) 
• The phase difference of a wave, denoted as Δ φ is the change in the state of vibration of particles in the medium about its mean position, Δ φ=2πΔ x/λ  
• The wave velocity is denoted as v and its respective equation is v=λ/T=fλ. It is the product of the wavelength and frequency of the propagation of the wave through the medium. The velocity is high at the mean position and zeroes everywhere else. The velocity of the medium through which the wave propagates and the velocity of the particles of the waves are not the same. It is measured in Hertz (Hz) 

Practice Problems 

1) The average range of frequencies at which humans can detect sound is between the range of 20 Hz to 20 kHz. Calculate the wavelength of the sound wave in these mentioned limits. The speed of sound in air is 340 m/s. 

Ans.

Here frequencies are given and the respective wavelengths are needed to be calculated. The speed of the wave is equal to the product of its wavelength and frequency. The formula used is$u=\nu \lambda$

For the minimum frequency 20 Hz the corresponding wavelength is

For maximum frequency 20 KHz the corresponding wavelength is

The wavelengths of the sound waves in the given limits are 0.017 m and 17 m.

2) A man watched a duck moving in the water. He noticed that the duck moved back and forth 20 times/minute. The man approximately measured the wavelength of the water to be around 1.4m. Determine the time taken by the duck to one-time front and one-time back. Also, calculate the velocity of the water. 

Ans.  Time period is defined time required to complete one back and forth motion. There are 20 back and forth motions accomplished in 1 minute. The time taken to complete 1 cycle of motion is,

20 times motion → 1 minute

1 time motion     → 1/20 minutes

                          → 0.05 minutes

                          → 0.05x60 seconds

                          → 3 seconds

Frequency is defined as the number of oscillations per unit time or it is the reciprocal of time period. It is calculated that time to complete one oscillation is 3 seconds.

The speed of the wave is product of the frequency and wavelength. The formula used

Context and Applications 

This topic is significant in the professional exams for both undergraduate and graduate courses, especially for 

• Bachelors in Science (Physics) 
• Masters in Science (Physics)