What is meant by relative motion?

It is a measurement of an object with regards to the other object which is moving or stable or stationary. For example, if we are traveling in a bus and the bus is moving at speed of 50km/hr, then our speed is according to the nearby passenger on the bus is zero. According to them, we are not moving. But if one person standing on the ground or road observes us from outside of the bus, according to him we are moving at a speed of 50km/hr and the bus is also moving at a speed of 50km/hr. Now, the motion is recognized by the observer, it depends on a frame of reference or observer. This is motion called relative motion.

What is meant by relative velocity?

A relative velocity is a velocity of a body A regarding the other body B or the pace of progress of relative situation of an item A concerning object B is called relative velocity.

Allow us to consider a two moving object A and B having velocity $v_a$ and $v_b$ concerning their casing of reference that is fixed stage or ground.

The velocity of object A relatives to the object B is given by,

$v_{ab}=v_a-v_b$           ...... (1)

Similarly, the velocity of object B relatives to the object A is given by,

$v_{ba}=v_b-v_a$           ....... (2)

From equations (1) & (2), we get,

$v_{ab} = - v_{ba}$

Henceforth, the magnitudes of both relative velocities are equals to each other.

$v_{ab}=v_{ba}$

The relative velocity may be expressed as in the form of a vector field,

In vector forms,

$\overrightarrow{v_{13}}=\overrightarrow{v_{12}}+\overrightarrow{v_{23}}$

$\overrightarrow{v_{13}}=\overrightarrow{v_{12}}+\overrightarrow{v_{23}}$

Here,

$\overrightarrow{v_{13}}$ is a final velocity or velocity with respect to the vector of objects A and C.

$\overrightarrow{v_{12}}$ is a velocity with respect to point A and B.

$\overrightarrow{v_{23}}$ is a velocity with respect to point A and C.

Dimension unit of a relative velocity

The dimension unit of a relative velocity is similar to the unit of velocity may be expressed as,

$M^0{L}^1{T}^{-1}$

Relative velocity in one dimension

In classical physics, to describe the position of any point in space, three variables are required. One dimensional motion is a simple system, in which only one variable is needed to describe the position of a point. If an object is moving with some velocity, which means the position of the object is changed with respect to time. If an observer needs to find the velocity, then the observer should find the position at each instant of time. The coordinated use to detect the position is called frame of reference.

The fundamental principle of relative velocity is that motion is not absolute, which means a motion may not be the same for all. The motion or velocity in particular depends upon the frame of reference. In other words, the observed velocity may be different for different observers.

If the motion of an object is one-dimensional then any one variable is required to specify the position of the object. Suppose an observer from the footpath at rest observes a car moving with a velocity of 30 km/s. There is also another observer who is observing the car from a bicycle which is moving with 10 km/s towards the car and the car is moving towards the bicycle. The first observer says the car is moving at 50 km/s but to the second observer, the car appears moving faster than 50 km/s.

The velocity of the car with respect to the first observer is v=50 km/s.

The velocity of the second observer is v’=10 km/s.

Since the car and the bicycle are moving in the same lane, the problem can be treated as one-dimensional. There are three different frames of reference in this problem.

• The rest observer: For this observer the car is moving at 50 km/s and the bicycle is moving 10 km/s.
• The observer in bicycle: For this observer the car is moving at the speed (50+10) km/s and the first observer is moving at 10km/s in opposite direction.
• The observer in car: For this observer the car is at rest, but the first observer is moving at 50 km/s and the bicycle is moving with velocity (50-10) km/s.

The velocities (50+10) km/s and (50-10) km/s are the relative velocities of the two bodies with respect to each other.

Relative velocity in two dimensions

Now, let us consider two objects M and N travel with a uniform velocity of v₁ and v₂ across the parallel line in the same direction. At the initial position, the time (t) is zero, and the displacement from their original position is denoted by x₁ and x₂ respectively.

After the displacement, the time becomes (t) and the displacement becomes x₁’ and x₂’ with respect to their original position, now the object M is denoted by,

$x_1’= x_1+ v_{1}t$     ...... (3)

In similarly, for object N is,

$x_2’= x_2+ v_{2}t$    ...... (4)

Now subtracting the equation (3) and (4), we get

$(x_2’- x_1’)=(x_2- x_1) + (v_2- v_1) t$     ....... (5)

Since x₁ and x₂ are initial displacements of an object N with respect to M at the time (t=0), now the equation may express as,

$x_0=(x_2- x_1)$    ....... (6)

Now substitute the value of equation (5) in equation (6), we get,

$(x_2’- x_1’)=\left(x_0\right) + (v_2- v_1) t$    ...... (7)

But, (x₂’- x₁’) is the relative displacement of an object N with respect to object P at the time (t), so the equation (7) becomes,

$x= x_0 + (v_2 – v_1) t$

Rewrite the above equation,

$(x - x_0)t= (v_2 – v_1)$

We can calculate the change in displacements per unit time by using the above relation. Here, the LHS is equal to RHS.

Relative velocity of an object moving with same direction

The relative velocity of an object N with respect to object M moving with the same direction, so the equation (6) becomes,

$v_{NM} = v_2– v_1$

Relative velocity of an object moving with opposite direction

The relative velocity of an object N with respect to object M moving with the opposite direction, so the equation (6) becomes,

$v_{NM} = v_2 + v_1$

Galilean transformation

From the above example, it is evident that a frame of reference is important to observe the motion in a systematic manner. While transforming from one frame of reference to another certain rules has to be followed. There are different transformation rules but the Galilean transformation is the simplest set of rules.

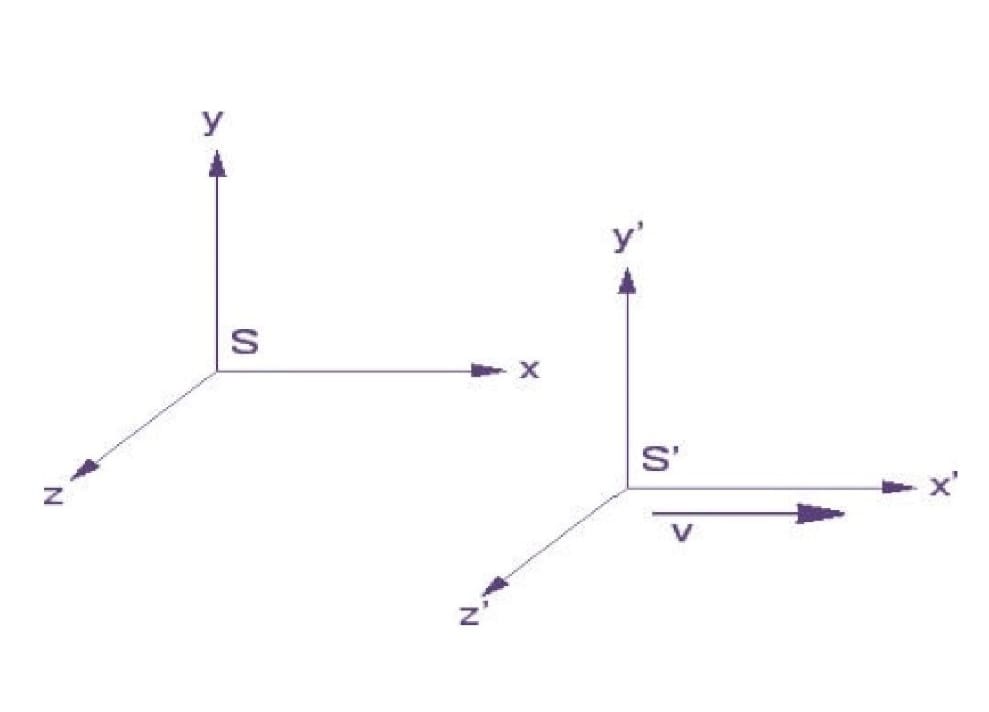
Let us take two frames of reference to suppose one frame at rest and the other one is moving at velocity v with respect to the first frame. Let (x,y,z,t) be the coordinates of the first frame and (x’, y’,z’,t’) be the coordinates of the second frame.

If the second coordinate is moving with velocity v from the first along x-direction then two coordinates can be related using the following equations,

$x=x’-vt$ 

$y=y’$

$z=z’$

$t=t’$

Lorentz transformation

The Galilean transformation is an accurate rule that is used to transform from one coordinate system to another. According to this rule, time is absolute which means time is the same for all the observers irrespective of their motion. But the important prediction of the special theory of relativity is that time is not the same for all the observers it depends upon the motion of the observers This effect is only observed when objects travel at a velocity close to the velocity of light called relativistic velocity. Hence, the Galilean transformation rules are not applicable for the objects moving with relativistic velocity.

Consider two frames of references S and S' with coordinates (x,y,z,t) and (x',y',z',t') respectively. Let the frame S' travel with relativistic velocity v along positive x-direction. According to the Lorentz transformation rule the two frames are related as follows,

$x\text{'}=\frac{x-vt}{\sqrt{1-{\displaystyle \frac{v^2}{c^2}}}}$

$y\text{'}=y$

$z\text{'}=z$

$t\text{'}=\left(\frac{1}{\sqrt{1-{\displaystyle \frac{v^2}{c^2}}}}\right)\left(t-\frac{vx}{c^2}\right)$

where c is the velocity of light.

Formula

The relative velocity may be expressed in the form of a vector field as,

$\overrightarrow{v_{13}}=\overrightarrow{v_{12}}+\overrightarrow{v_{23}}$

Context and Applications

This topic is one of the important applications in all forms of classical mechanics and it is significant for both undergraduate and postgraduate courses, especially for bachelors and masters in science (physics).

Practice Problems

Question 1: A bus traveling with a velocity of 50 km/hr in the south direction. It experiences a wind travels in the west direction at a rate of 15 km/hr. Now calculate the final velocity of a bus.

1. 15.5 km/hr
2. 52.20 km/hr
3. 20.52 km/hr
4. 18.12 km/hr

Answer: The correct option is b.

Given data:

The velocity of the wind (V_w) is 15 km/hr.

The velocity of a bus (V_a) is 50 km/hr.

Explanation: Now the relative velocity of a bus with respect to the earth is given as and the angles between the velocity of the wind and the bus are 90º.

Now using a Pythagorean theorem, the final velocity may be calculated as,

$v_f=\sqrt{({v_a}^2+{v_w}^2)}$

$$\begin{gathered} {v_f}^2={\left(50\right)}^2+{\left(15\right)}^2 \\ {v_f}^2=\left(2500\right)+\left(225\right) \end{gathered}$$

${v_f}^2=2725$

$v_f=\sqrt{2725}$

$v_f=52.20$

Henceforth, the final velocity of a bus is 52.20 km/hr.

Question 2: An object is moving with a stationary frame, this type of motion is called as ________.

1. Relative motion
2. Absolute motion
3. Circular motion
4. Parabolic motion

Answer: The correct option is b.

Explanation: The motion with respect to a stationary frame is called absolute motion.

Question 3: Suppose two objects are moving in an adverse direction with non-zero velocity, which of the following statement is true?

1. Relative velocity is greater than the absolute velocity
2. Relative velocity is lesser than the absolute velocity
3. Relative velocity equals to the absolute velocity
4. None of the above

Answer: The correct option is a.

Explanation: We know that the relative velocity may be expressed as,

$\overrightarrow{v_R}=\overrightarrow{v_A}-\overrightarrow{v_B}$

When both the velocities are in an adverse direction, the equation becomes,

$$\begin{gathered} \overrightarrow{v_R}=\overrightarrow{v_A}-(-\overrightarrow{v_B}) \\ \overrightarrow{v_R}=\overrightarrow{v_A}+\overrightarrow{v_B} \end{gathered}$$

Hence, from this equation, the magnitudes of the relative velocity are increased. Therefore, the relative velocity is greater than the absolute velocity.

Question 4: A man is sitting on a bus moving with a constant velocity, the man sees the things surrounding him, in the _________.

1. Absolute frame of reference
2. Ground frame of reference
3. Relative frame of reference
4. None of the above

Answer: The correct option is c.

Explanation: A man or observer is not in the stationary position with respect to the ground. The observer is in +a stationary position with respect to the frame of the moving bus i.e. the observer is stationary with respect to the relative frame of reference.

Question 5: An object A is moving in the east direction, while another object B is moving towards the west direction. The velocity of A is greater than B. If east is taken as the positive direction, which of the following relative velocity is positive?

1. Velocity of B with respect to A
2. Velocity of A with respect to B
3. Velocity of A with respect to ground
4. None of the above

Answer: The correct option is b.

Explanation: We know that the relative velocity may be expressed as,

$\overrightarrow{v_R}=\overrightarrow{v_A}-\overrightarrow{v_B}$

The velocity A is greater in magnitudes hence the velocity of A becomes positive.