What is a Plano-Convex Lens?
To understand the topic well we will first break down the name of the topic, ‘Plano Convex lens’ into three separate words and look at them individually.
Lens: Lens is just a piece of transparent material, generally glass, which has at least one curved surface. It is a transmissive optical device used for either concentrating the lay rays on one point or for dispersing the light rays. Lens function based on the principle of refraction. There are six different types of lenses. Each type of lens has two focal points Unlike mirrors, at least one surface of a lens must be curved if not both
Convex: Convex lens, also known as a converging lens, is used to bend the parallel light ray incident on it so that they converge at a spot beyond the lens. This spot is termed the focal point. These are most commonly used in eyeglasses to correct farsightedness.
Plano: Plano has a dictionary meaning of a flat level.
Now if we combine these three individual word’s definitions, we can obtain our definition for plano convex lens which is as follows –
A plano convex lens has a structure wherein one side of the lens is curved outwards and the other side is plane. It is used to converge parallel light rights onto one point which is the positive focal point.
In this process, it forms real images that can be manipulated by the use of spatial filters.
The structure is such that it is an asymmetrical lens. This feature of a plano convex lens helps reduce the spherical aberration in instances where the object and the image are no at equal distances from the lens.
The Shape of a Plano Convex Lens
The positive focal length elements of the lens have one curvature surface and one flat surface. The curved surface has a focusing effect on the rays of light but the flat surface lacks this feature. They come in varied mm diameter.
Image Formed
To obtain an image with maximum sharpness the curved side of the lens should be facing toward the object and not the flat side. This quality of the image is determined based on its orientation and two positive focal lengths.
If the lens is set up such that the light ray enters and exits from the curved surface of the lens, you will obtain low spherical aberration. The case where you will receive optimum reduction is when the object is placed at infinity.
Uses
These lenses are used in various industries like robotics, defence, and medicine.
Some of the uses include laser cutting, monochromatic process, and light collimation.
They are most commonly used when the object is far away from the lens. In optical physics, it is termed infinite conjugate. An example of the same is when you want to focus light from a faraway object like the star.
Types of Lens
N-BK7: These lenses are the perfect choice for general-purpose visible and NIR applications, including high homogeneity and low bubble and inclusion content.
UV Fused Silica lenses: these are recommended for UV rays or higher energy applications, combining a very low thermal expansion coefficient and excellent transmittance.
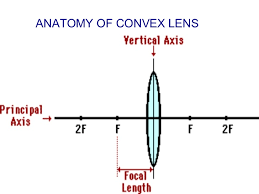
Anatomy of Lens Diagram
Principal axis: it is a horizontal straight line passing through the centre of the lens.
Optical axis or vertical axis: A vertical line passing through the centre of the curvature of a lens.
Optical centre:This is the central point of the lens. Where the principal and optical axis intersect.
Focal point (F): It is a point on the principal axis at which the parallel rays converge.
Focal length: It is the distance between the center of the convex lens and the focal point.
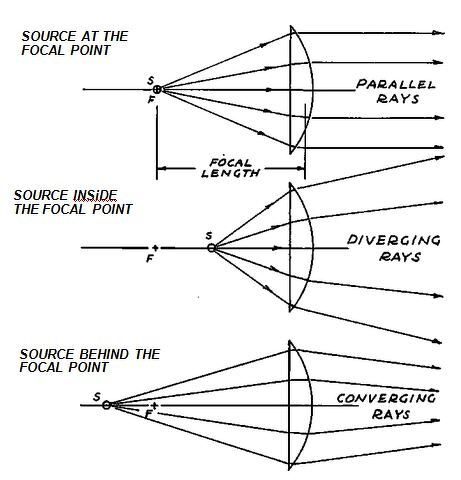
Real Image
When the image formed is inverted as compared to the object, the image formed is called a real image. This type of image can be captured on a screen. A converging lens produces a real image when the object is placed at a point more than one focal length from the lens.
Virtual Image
When the image formed is upright as compared to the object, the image formed is called a virtual image. These images cannot be captured on a screen. A converging lens will produce a virtual image when the object is placed within one focal length of the lens. It produces a magnified image on the same side of the lens as the object. It acts as a magnifying glass.
Magnification of Convex Lens
It is the ratio between the height of the image and the height of the object.
m = hi ÷ ho
where m = magnification, hi = height of object and ho = height of object
If the value of m is one, the size of the image and object are the same. If the value of m is greater than one, the image is enlarged. And if m is less than one, the image is diminished.
If there is a positive magnification, the image formed is virtual but if the magnification is negative the image formed is a real image.
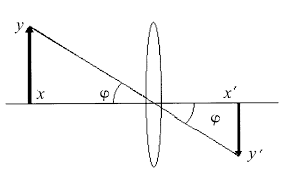
Ray Diagram
We can draw a ray diagram for a plano convex lens as follows
- A ray from the top of the object passing through the optical center without a change in direction.
- A parallel ray from the top of the object till the optical axis. It then refracts to pass through the focal point on the other side of the lens.
- A ray passing through the focal point to the optical axis. It then refracts to form a ray parallel to the principal axis.
The point where these three rays intersect is where the image is formed.
However, you can obtain the position of the image by drawing any two of the three rays mentioned above. The point at which the two rays will intersect will be the top of the image. Three rays are drawn for greater accuracy of the position of the image.
The properties of the image formed depend on the placement of the object on the principal axis. The table summarizes the properties:
| Location of the object (left of lens) | Location of image formed (right of lens) | Properties of the image formed |
| At infinity | At F | It is a real image. It is inverted and diminished |
| Beyond 2F | Between F and 2F | It is a real image. It is inverted and diminished |
| At 2F | At 2F | It is a real image. It is inverted and the same size as the object |
| Between F and 2F | Beyond 2F | It is a real image. It is inverted and enlarged. |
| At F | At infinity | It is a real image. It is inverted and highly magnified. |
| Between F and optical centre | On the same side of the lens as the object | It is a virtual image. It is erect and enlarged. |
Thin Lens Equation
Where f is the focal length
v: the distance that lies between the image and the optical centre of the lens
u: the distance that lies between the object and the optical center of the lens The focal length for convex lenses is always positive.
Calculate the Focal Length of the Lens
Where f is equal to the focal length
Rf is equal to the radii of the curvature of the front surface
Rb is equal to the radii of the curvature of the back surface
For a plano convex lens, Rf is greater than zero while Rb is equal to infinity
Guidance
- You should try remembering the table of the object positions and image properties.
- While drawing ray diagrams it is important to be precise and draw thin lines to get an accurate position of the image.
- Prefer drawing three rays on ray diagrams for accurate results.
Similar Topics
- The concave or diverging lens
- Converging lens
- Mirrors and lenses
- Refraction
- Optical physics
Context and Applications
This topic is studied in class 11th, 12th and Bachelors in Science (Physics).
Want more help with your physics homework?
*Response times may vary by subject and question complexity. Median response time is 34 minutes for paid subscribers and may be longer for promotional offers.
Plano-Convex Lens Homework Questions from Fellow Students
Browse our recently answered Plano-Convex Lens homework questions.