What is the maximum power transfer theorem or MPTT?

A resistive load will consume maximum power from a network when the load resistance is equal to the resistance of the network as viewed from the open-circuit terminals, with all energy sources removed leaving only the internal resistances. Physicist Moritz von Jacobi introduced the maximum power transfer theorem in 1940. Therefore, this theorem is also known as Jacobi's law.

Although this theorem is important for all branches of electrical engineering, it has limited applications in power distribution and transmission as it requires maximum efficiency and maximum power transfer. This theorem has 50% efficiency that is enough for communication networks because in these types of networks maximum power transfer is required with less priority to efficiency. In the operation of transmission lines and antennas, the problem of MMTT is crucial.

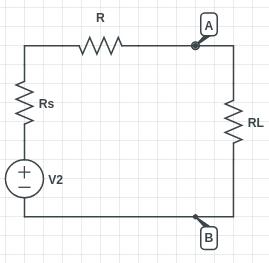
In the above circuit diagram, a load resistance R_L is connected across terminals A and B of a network consisting of a voltage supply V₂, its internal resistance R_s and internal resistance of connecting wires R. Let us assume R_i to be the sum of R_s and R i.e. total internal resistance or source resistance of the circuit after excluding load resistance R_L.

According to the maximum power transfer theorem, R_L will consume maximum power from the network when R_L=R_i

Proof

Applying KVL in the circuit diagram above, we get

$E=I(R_L+R_i)$

Circuit current

$I=\frac{E}{R_L+R_i}$

Power consumed by the load is

$P_L=I^2{R}_L=\frac{E^2{R}_L}{{(R_L+R_i)}^2}$

For P_L to be maximum,

$\frac{d{P}_L}{d{R}_L}=0$

Differentiating the above equation, we get

$$\begin{gathered} E^2\left\{\frac{{(R_{th}+R_L)}^2\times 1-R_L\times 2(R_{th}+R_L)}{{(R_{th}+R_L)}^4}\right\}=0 \\ \Rightarrow {(R_{th}+R_L)}^2-2R_L(R_{th}+R_L)=0 \\ \Rightarrow (R_{th}+R_L)(R_{th}+R_L-2R_L)=0 \\ \Rightarrow (R_{th}-R_L)=0 \\ \Rightarrow R_{th}=R_L \end{gathered}$$

∴ Maximum power is $P_{max}=\frac{E^2{R}_L}{4{R_L}^2}=\frac{E^2}{4R_L}=\frac{E^2}{4R_{th}}, \sin ce R_{th}=R_L$

Power transfer efficiency

If P is the power supplied to the load and P_t is the power supplied by the voltage source, then power transfer efficiency is given by $\eta =\frac{P}{P_t}$

The voltage source V₂ provides power to both internal resistance R_i and load resistance R_L.

The above diagram shows both $\eta$ and $P_{max}$ in one graphical plot.

Efficiency $\eta$ tends to be unity when R_L=∞ and it has value of 0.5 when R_L=R_i . This means at maximum power transfer the efficiency is 50%. In a communications system, this efficiency holds less importance as compared to a power system.

Importance of impedance matching

In case of mismatch of impedance between source impedance and load, the impedance will make the signal reflect the source causing destructive interference leading to reduced power transfer to the load. Impedance matching is used to design source to avoid this along with load impedances to reduce signal reflection and maximize power transfer. In DC circuits the source resistance and load resistance should be equal. In AC circuits the source impedance should be equal to either load impedance or complex conjugate of load impedance.

Maximum power transfer theorem in AC circuit

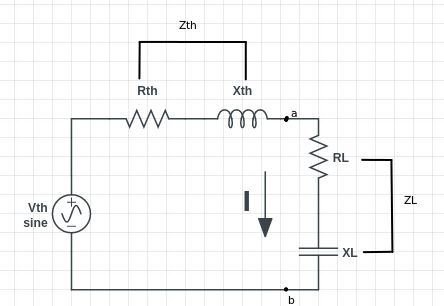
In the case of AC circuits, the internal impedance is (R₁+jX₁), and load impedance is (R_L+jX_L). In this case, the maximum power transfer (P_max) will happen when the modulus of load impedance is equal to the modulus of internal impedance, i.e. |Z_L|=|Z₁|

In the case of variable load resistances, the maximum power transfer is obtained when the load impedance is the complex conjugate of internal impedance. For example, if the internal impedance is (R₁+jX₁), then for maximum power transfer the load impedance has to be (R₁-jX₁)

In AC circuits, maximum power transfer is achieved when load impedance (Z_L) is equal to Thevenin equivalent impedance (Z_th)

If Z_th=R_th+jX_th 

then, Z_L for max power = R_th-jX_th

This means if the Thevenin impedance includes an inductor then the load impedance  should have a capacitor for maximum power transfer condition as shown in the circuit diagram.

$I=\frac{E_{th}}{Z_{th}+Z_L}=\frac{E_{th}}{(R_{th}+R_L)+j(X_{th}-X_L)}$

For maximum power transfer, X_th should be equal to X_L.

∴$\frac{E_{th}}{(R_{th}+R_L)+j(X_{th}-X_L)}=\frac{E_{th}}{(R_{th}+R_L)}$

For maximum power transfer, R_th should also be equal to R_L.

∴$$\begin{gathered} \frac{E_{th}}{(R_{th}+R_L)}=\frac{E_{th}}{2R_{th}} \\ {P}_L={I_L}^2{R}_L={\left(\frac{E_{th}}{2R_{th}}\right)}^2{R}_L=\frac{{E_{th}}^2}{4{R_{th}}^2}{R}_{th}=\frac{{E_{th}}^2}{4R_{th}}=P_{max} \end{gathered}$$

Example question

In the given network, find the value of load resistor R_L such that the maximum possible power will be transferred to R_L. Using the maximum power transfer theorem, find also the value of the maximum power and the power supplied by the source under these conditions.

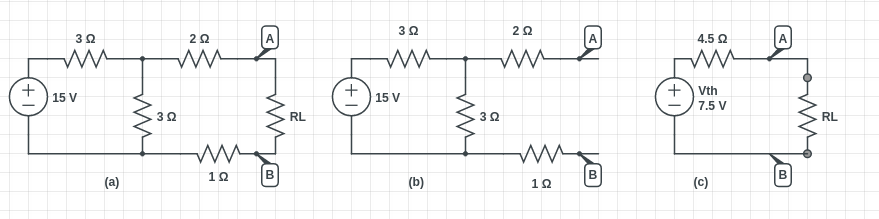
Looking at figure a, we need to remove the R_L and find the Thevenin equivalent  source for the circuit to the left of terminals A and B. 

In figure b, V_th is equal to the drop across the vertical resistor 3Ω because current will not flow through the 02Ω and 1Ω resistors. Drop across each 3Ω resistor is given by,

$V_{th}=\frac{15}{2}=7.5V$ , also known as the Thevenin voltage.

If we short circuit the 15V supply, then Thevenin resistance is given by,

$R_{th}=2+1+\left(3\right|\left|3\right)=4.5\Omega$

According to the maximum power transfer theorem, maximum power will take place when R_L=R_th=4.5Ω

Maximum power drawn by R_L is

$\frac{2V_{th}}{4}\times R_L=\frac{7.52}{4}\times 4.5=3.125W$

Same amount of power is also developed in R_th, hence,

$power supplied by source=3.125\times 2=6.25W$

Context and Applications

The concept of maximum power transfer theorem is a fundamental of electrical network analysis and its knowledge is crucial in various diploma, undergraduate, and graduate degrees like:

• Bachelors of Technology (Electrical Engineering)
• Masters of Technology (Telecommunication Engineering)
• Bachelors of Science (Physics)

Practice Problems

Q1. To what does the application of Thevenin's theorem in circuit analysis lead?

1. An ideal current source
2. An ideal voltage source
3. A voltage source with impedance in series
4. A voltage source with impedance in parallel

Answer: c

Explanation: To find Thevenin equivalent in a circuit, we need to open the circuit, load terminals keeping the voltage source and impedance/resistance in series.

Q2.  What should be the value of load resistance R_L, if the Thevenin resistance is R_th, then to achieve maximum power?

1. 0
2. 1
3. R_th
4. ∝

Answer: c

Explanation: According to the maximum power transfer theorem, maximum power dissipation happens when the Thevenin resistance R_th is equal to load resistance R_L.

Q3. What is the maximum efficiency at maximum power transfer in an electrical system?

1. 30%
2. 50%
3. 70%
4. 100%

Answer: b

Explanation: Maximum power transfer to load resistance happens at 50% efficiency.

Q4.  What is required to find out what value of load resistance leads to maximum power dissipation?

1. Open-circuit the load resistance terminals and find out the internal resistance of the circuit.
2. Short-circuit the load resistance terminals and find out the internal resistance of the circuit.
3. Find the sum of load resistance and internal resistance of the other elements.
4. Find the sum of current going in and out of the load resistance.

Answer: a

Explanation: To find the load resistance value for maximum power dissipation, we need to open-circuit the terminals across load resistance and then find the internal resistance of the circuit.

Q5. How does reactance differ from impedance?

1. Reactance is the sum of impedance and resistance.
2. Impedance is from inductors and reactance is from capacitors.
3. Reactance is the sum of resistance and impedance.
4. Impedance is the sum of reactance and resistance.

Answer: d

Explanation: Reactance is the sum of the combined resistive effect of the inductor and capacitor in an AC circuit. Impedance is the sum of resistance and reactance. DC circuit does not have reactance, they only have resistance. The maximum power transfer theorem applies to both the AC circuit and DC circuit.

Related Concepts

• Thevenin's theorem
• Superposition theorem
• Voltage and current sources
• AC Network analysis