What is the composition of atomic nuclei ?

The ratio of protons to neutrons inside an atomic nucleus governs its stability. An atomic nucleus contains positively charged protons and neutral neutrons, typically packed within a nuclear radius of order of 10^-15 metres. Z represents the total number of protons, whereas A represents total number of nucleons (sum of number of protons and neutrons inside atomic nucleus) of an atomic nucleus, then the nucleus of the element is represented symbolically as ${}_Z{}^{A}X$ .

${}_1{}^{1}H$ represents a hydrogen nucleus that has 1 proton and 0 neutrons in its atomic nucleus.

${}_{83}{}^{209}Bi$ represents bismuth nucleus, that has 83 protons and 209-83 = 126 number of neutrons in its nucleus.

Nuclei that contain the same number of protons, but different number of neutrons are known as isotopes. Carbon element exists in two isotopes forms: 98.9% of carbon atoms are of the form ${}_6{}^{12}C$ (6 protons + 6 neutrons), whereas 1.1% of carbon atoms are of the form ${}_6{}^{13}C$ (6 protons + 7 neutrons).

What decides the stability of the atomic nucleus?

Protons repel each other with long-range electrostatic forces between each other. It’s the strong binding nuclear forces between nuclear particles that hold the atomic nucleus together. Nuclear forces are short range, strongly attractive and charge independent. For a nucleus to be stable, the electrostatic repulsion is balanced by the attraction between the nucleons. But one proton can repel all the protons in the nucleus, as electrostatic forces are long range. On the other hand, a proton or a neutron can attract only its immediate neighbors with nuclear forces as these are short range forces. With the increase in the number of protons the number of neutrons in the nuclei has to increase in order to maintain the stability of the atomic nucleus. There comes a point when the large number of neutrons no longer achieves the balance of electrostatic and nuclear forces.

The stable nucleus with large number of protons is Z = 83 is that of bismuth ${}_{83}{}^{209}Bi$ which contains 126 neutrons. All nuclei with number of protons in excess of 83 are seen as unstable. These nuclei spontaneously decay or rearrange their internal structures with time to achieve greater stability. This spontaneous disintegration of atomic nucleus causing rearrangement of internal structures is called radioactivity. This phenomenon was first discovered by the French physicist, Henry Becquerel in 1896.

Radioactivity

When an unstable or radioactive nucleus disintegrates spontaneously, certain kinds of particles or rays or high-energy photons or radiation are released. These rays produced by naturally occurring radioactive nuclei are alpha (α) rays , beta (β) rays and gamma (γ) rays. 

α rays consist of positively charged particles, each one being the ${}_2{}^{4}He$ nucleus of helium. β rays consist of negatively charged electrons along with an energy particle neutrino. γ rays are high energy electromagnetic radiations that do not cause a transmutation of one element into another. Its release simply results in change of energy levels of the nucleus.

Radioactive Decay

In a group of radioactive sample of nuclei, individual disintegration occurs randomly. As time advances, the number N of parent nuclei decreases in a given sample.

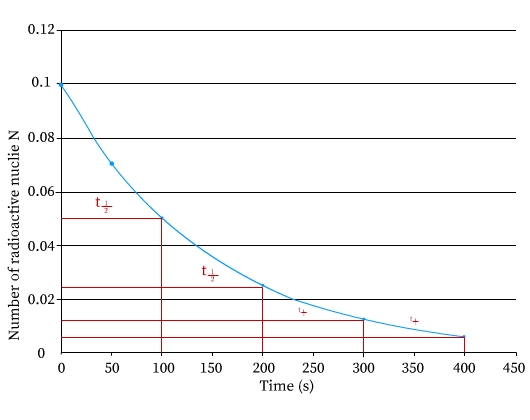
The figure shows a graph between number of radioactive nuclei N along y axis versus time in seconds along the x axis. The plot shows an exponential fall in the number of radioactive nuclei with time.

This graph of N versus time T indicates that the decrease occurs in smooth fashion. Number of atoms N approaches to 0 after enough time has elapsed.

Half-life, T_1/2 time of radioactive nuclei is defined as time required by one-half of the number of parent radioactive nuclides to decay by half in number or reduce to half the number.

For example, radioactive Radium element, ${}_{88}{}^{226}Ra$ disintegrates into half of its original number in about 1600 years. In another 1600 years, another half the numbers will disintegrate, leaving only one fourth of the original numbers intact. So if in the above graph, if at time T = 0, the number of radioactive nuclides are N_o, after time = T_1/2 , the number present is N = N_o/2. Further at time = 2T_1/2, the number present will be N = N_o/4 .

The value of half-life of radioactive nuclides depends upon the nature of the sample. It may vary from few seconds to billions of years.

The activity of radioactive sample is the number of disintegration per second that occur in a given sample of radioactive sample. But note that each time a disintegration occurs the number N of remaining radioactive nuclei decreases. Activity of a radioactive sample is defined by dividing the change in number of radioactive nuclei, ∆N by time interval ∆t during which the change occurs. Average activity over the time interval ∆t is given as ∆N/∆t and this is proportional to the number of radioactive nuclei remaining in the sample.

$\frac{∆N}{∆t} \alpha -N$

$\frac{∆N}{∆t} = -\lambda N$

Here λ is proportionality constant, referred to as decay constant of the radioactive sample. The negative sign here indicates that with time due to subsequent disintegrations, the number of radioactive atoms decreases subsequently.

The SI unit of activity is called Becquerel (Bq), where 1 becquerel of activity is equal to 1 disintegration per second. A bigger unit of activity of radioactive nuclei is curie (Ci) where

1 Ci = 3.7 x 10¹⁰ Bq

For an estimate of activity of commonly used radioactive radium:

The activity of radium used in watch dial to make it glow in dark is about 4 x10⁴ Bq , that’s purely harmless to human exposure.

On the other hand, the activity used in radiation therapy for cancer treatment is approx. billion time greater, or 4 x 10¹³ Bq! Such an exposure for a fraction of seconds causes irreversible effect on living tissues.

For the graph in Fig 1, depicting the radioactive decay of a radioactive sample, the equation that governs the rate of fall in number of radioactive nuclei as a function of time is given as:

$N = N_0 e^{-\lambda t}$, assuming that at time t = 0, the number of radioactive nuclei were N_o

Applying the conditions that at t = T_1/2, number of nuclei present, N=N_o/2, the above equation is reduced to

$T_{\frac{1}{2}} = \frac{\ln 2}{\lambda } = \frac{0.693}{\lambda }$

Radioactive Dating

An important application of radioactivity is the determination of the age of archeological samples. If the fossil sample contains radioactive nuclei when it was formed, then the decay of the nuclei marks the passage of time as half of the radioactive sample decays after every some time equal to its half life.

If the half-life of the radioactive element present in the sample is known, a measurement of the present day number of radioactive nuclei relative to original number gives an estimate of the age of the fossil sample.

Since the activity of the sample is proportional to rate of disintegration, one way to know the age of the sample is to compare the present day activity with initial activity. Mass spectrometer is deployed to know the present day number of radioactive nuclei in a sample.

Carbon Dating

${}_6{}^{14}C$ isotope of carbon is radioactive with half-life of 5730 years. In earth’s atmosphere, it is present in the ratio of 1 atom for every 8x10¹¹ atoms of normal non-radioactive carbon ${}_6{}^{12}C$. This ratio is maintained as for every loss due to radioactive decay of ${}_6{}^{14}C$ is offset by the creation of new ${}_6{}^{12}C$ atoms due to cosmic ray interaction with upper layers of atmosphere. The same ratio of ${}_6{}^{14}C$and ${}_6{}^{12}C$ is maintained inside all living organisms as long as they are alive. But as soon as an organism dies, it no longer absorbs ${}_6{}^{14}C$ from the atmosphere, but the count of ${}_6{}^{14}C$ present inside its body begins decline due to radioactive decay.

So by applying the principles of radioactive decay rules to determine the present day number of ${}_6{}^{14}C$ numbers in the fossil remains, the age analysis of the organism can be done. This process of age analysis of fossil using radioactive ${}_6{}^{14}C$ is carbon dating.

Practice Problems

Q1. Carbon-radioactive isotope sample has 1000 nuclei. If its half-life is 5730 years, how many nuclei remain after 17190 years?

1. 500
2. 250
3. 125
4. 64

Answer: 125

Q2. During the disintegration of radioactive nuclei, the total energy, electric charge, linear momentum, angular momentum and nucleon number are necessarily conserved.

1. True
2. False

Answer: True

Q3. Out of three types of radiations, alpha, beta and gamma rays, emitted by the unstable radioactive nucleus, only the …………………… radiations fail to get deflected by the magnetic fields.

a. alpha

b. beta

c. gamma

Answer: gamma

Q4. Radioactivity can be induced by applying strong electrical and magnetic fields on a heavy atomic nuclei.

1. True
2. False

Answer: False

Q5. During radioactive decay the number of nuclei in the sample decreases ………………………….. with time.

   a. exponentially

  b. linear

Answer: exponentially

Context and Applications

The concept of Half Life has numerous practical applications some of them includes figuring out the effective drug dosage to finding out when a radioactive becomes safe to use. If you look for a career in Nuclear Physics you will need this concept.

This topic is significant in the professional exams for both undergraduate and graduate courses, especially for 

• Bachelors in Science (Physics) 
• Masters in Science (Physics)