
Consider the two-loop circuit shown in Fig P7.1. The currents
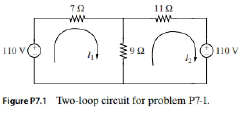
(a) Find
(b) Write the system of equations
(c) Find
(d) Find
(a)
The value of
Answer to Problem 1P
The value of
Explanation of Solution
Given:
The first system equation is:
The second system equation is:
Calculation:
Solve the system equation (1) for
Simplify further.
Substitute
Rearrange for
Substitute
Conclusion:
Thus, the value of
(b)
The system of equation in the form of
Answer to Problem 1P
The matrix form of the equation is
Explanation of Solution
Concept used:
The system of two simultaneous equations of variables in the form
Here,
Calculation:
The system of equations represented by equation (1) and equation (2) can be written in the matrix form as follows:
Conclusion:
Thus, the matrix form of the equation is
(c)
The value of
Answer to Problem 1P
The value of
Explanation of Solution
Concept used:
Write the expression to calculate the value of the current using matrix method.
Here,
Write the inverse of the matrix.
Here,
Calculation:
The equation in the form
The determinant of matrix
The adjoint of matrix
Substitute
Substitute
Conclusion:
Thus, the value of
(d)
The value of
Answer to Problem 1P
The value of
Explanation of Solution
Concept used:
Write the expression for
Here,
Write the expression for
Here,
Calculation:
Substitute
Substitute
Conclusion:
Thus, the value of
Want to see more full solutions like this?
Chapter 7 Solutions
Introductory Mathematics for Engineering Applications
Additional Math Textbook Solutions
Basic Technical Mathematics
Fundamentals of Differential Equations (9th Edition)
Advanced Engineering Mathematics
PRACTICE OF STATISTICS F/AP EXAM
Excursions in Modern Mathematics (9th Edition)
Calculus, Single Variable: Early Transcendentals (3rd Edition)

