What is meant by Angular acceleration?

Angular acceleration is defined as the rate of change in angular velocity with respect to time. It has both magnitude and direction. So, it is a vector quantity.

The angular acceleration is denoted by α.

The S.I unit of an angular acceleration is $rad/s^2$.

Types of angular acceleration

Angular acceleration is mainly classified into two types. They are:

• Spin angular acceleration.
• Orbital angular acceleration.

Angular acceleration formula

The angular acceleration might be expressed in the form of,

$\alpha = \frac{d\omega }{dt}$

In terms of angular displacement,

$\alpha =\frac{d^2\theta }{d{t}^2}$

or  $\alpha =a_{T}r$

Where,

ω is  angular velocity.

$a_T$ is  tangential acceleration or linear acceleration.

r is the radius of a rotating circular disc.

The above equation indicates linear acceleration and angular acceleration are directly proportional to each other. The linear acceleration is larger than the angular acceleration and vice versa.

Derivation of angular acceleration

Angular acceleration is a ratio between the change in angular velocity and the change in time. It is given by,

$\alpha =\frac{d\omega }{dt}$

Here,

α is  angular acceleration.

ω is  angular velocity.

t is the time.

We know that angular velocity could be given as,

$\omega =\frac{ v}{r}$

Angular velocity may be expressed in terms of change in angular displacement with the change in time.

$\omega =\frac{\theta }{t}$

Where,

θ is the angular rotation. 

t is the time.

Tangential acceleration

The tangential acceleration is described as the rate of change of tangential velocity of a body with respect to time, that is, moving in a spheroid or circular path.

The tangential acceleration is given as,

$a_t=\frac{∆v}{∆t}$

The tangential acceleration in terms of distance is,

$a_t=\frac{d^{2}s}{d{t}^2}$

Where,

$a_t$ is the tangential acceleration or linear acceleration.

∆v is the change in angular velocity.

∆t is the change in time.

Kinematic equation for angular acceleration

There are some special cases for rotational motion at constant angular acceleration. The approximation of kinematic equations are represented as:

$\omega ={\omega }_0+\alpha t$

$\theta ={\theta }_0+{\omega }_{0}t+\frac{1}{2}\alpha t^2$

${\omega }^2={{\omega }_0}^2+2\alpha (\theta -{\theta }_0)$

Average angular velocity formula

An average angular velocity is described as a ratio between the change in angular coordinates and the change in time.

Average angular velocity is expressed as,

$$\begin{gathered} {\omega }_{avg}=\frac{∆\theta }{∆t} \\ {\omega }_{avg}=\frac{{\theta }_2-{\theta }_1}{t_2-t_1} \end{gathered}$$

Where,

${\omega }_{avg}$ is a average angular velocity (rad/s).

∆θ is a change in angular co-ordinates (radian).

∆t is a change in time (sec).

What is meant by Angular displacement?

An angular displacement is described as the smallest angle between the starting point and the ending point of an object that undergoes circular motion with respect to a fixed point. It is a vector quantity and it has both magnitude and direction or the angle built by the object from its rest point to endpoint occurred by the rotational motion is known as angular displacement.

An angular displacement is denoted by θ.

The S.I unit of the angular displacement is radian or rad.

Angular displacement formula

An angular displacement can be expressed as,

Angular displacement =  ${\theta }_f-{\theta }_i$

Where,

$\theta =\frac{s}{r}$

θ is the angular displacement of an object.

s is a distance covered by an object in a circular path.

r is the radius of a circular path.

The angular displacement is calculated in terms of angular velocity as,

$\theta =\omega t+\frac{1}{2}\alpha t^2$

Where

α is a acceleration of an object.

ω is an angular velocity.

t is a time.

What is meant by angular speed?

Speed is the quantity that represents how fast or slow the objects are moving. Henceforth, the angular speed refers to how fast the object rotates in a circular path or itself.

An angular speed formula is used to calculate the distance covered by the object with respect to its rotation and/or revolution by the required time. The speed calculates how fast or slows the object moving and the angular speed calculates rotational motions of an object. The angular speed is a vector quantity, it has both magnitude and direction.

The angular speed is denoted as ω.

The S.I unit of angular speed is radians per second or rad/sec.

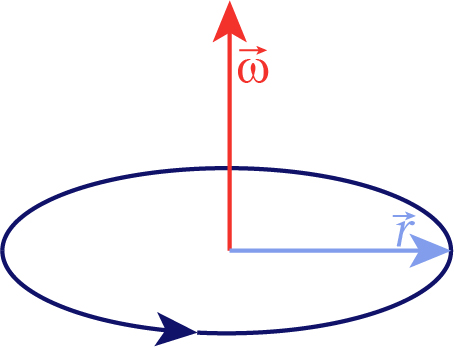
Angular speed formula

Angular speed is calculated using the formula below,

$Angular speed= \frac{Total dis\tan ce}{required time}$

$\omega =\frac{\theta }{t}$

Where,

ω is the angular speed in terms of radian/sec.

θ is the total distance covered by the object in terms of radians.

t is the required time to complete rotation in terms of seconds.

An angular speed for one complete rotation is,

$\omega =\frac{2\pi }{t}$

The S.I unit of angular speed is rad/sec.

Relationship between angular speed and linear speed

Let us taking an object which is moving in a circular path having a radius r and their angular displacement is θ. Here,

Angle (θ) = $\frac{Arc}{Radius}$

We know that the linear speed is,

$v=\frac{s}{t}$

Where,

s represents the linear displacement.

And

$\theta =\frac{s}{r}$

Now the linear speed is,

$$\begin{gathered} v=\frac{\theta r}{t} \\ v=r\omega \end{gathered}$$

Therefore, the angular speed is,

$\omega =\frac{v}{r}$

Here, v is the linear velocity.

This equation represents the relation between the linear speed, angular speed, and the radius of a circular path.

Examples

• At the point when quarterbacks toss the football, they embed a twist with their fingers, so the ball turns quickly as it flies through the air. Football fans characterize a decent pass as a tight winding.
• When we are shot, bullet that comes out of a gun barrel that has grooves cut into them to have a spin on them.

Context and Applications

This topic is one of the crucial applications in all forms of classical mechanics and it is significant for both undergraduate and postgraduate courses, especially for bachelors and masters in science (physics).

Practice Problems

Question 1: A dog is situated at the corner of a round-shaped rotating disc. The variation of angular velocity is 50 rad/s for 15 seconds. Calculate their angular acceleration.

$$\begin{gathered} a.2.41 rad/s^2 \\ b. 4.35 rad/s^2 \\ c.7.42 rad/s^2 \\ d.3.33 rad/s^2 \end{gathered}$$                                     

Answer: The correct option is (d).

 Given data:

The change in angular velocity is 50 rad/s.

The required time is 15 s.

Explanation:

We know that angular acceleration formula is,

$\alpha =\frac{d\omega }{dt}$

Where,

ω is the angular velocity.

t is time.

$\alpha =\frac{50}{15} rad/s^2$

$\alpha =3.33 rad/s^2$

Henceforth, the angular acceleration is $3.33 rad/s^2$.

Question 2: A circular wheel completes 180 revolutions per minute. What is the angular velocity of the wheel?

1.  2π rad/s                                                         
2.  6π rad/s
3. 4π rad/s   
4. 8π rad/s                                                      

Answer: The correct option is (b).

Given data:

The number of revolutions per minute is 180 RPM.

Explanation:

We know that,

$1RPM =\frac{2\pi }{60} rad/s$

For 180 RPM, the angular frequency may be expressed as,

$\omega =180x\frac{2\pi }{60}$

$\omega =6\pi rad/s$

Henceforth, the angular velocity is 6π rad/s.

Question 3: What is the unit of angular acceleration?

1. Radian per second
2. Degree per second
3. Radian per second square
4. None of the above

Answer: The correct option is (c).

Explanation:

We know that the angular displacement is denoted as θ in terms of radians.

An angular displacement between the two objects which revolves about an axis with respect to the time is known as angular velocity.

$\omega =\frac{d\theta }{dt}$

Angular velocity ω is described in terms of radian/s.

The rate of change of angular velocity is known as angular acceleration.

$\alpha =\frac{d\omega }{dt}=\frac{d^2\theta }{d{t}^2}$

Angular acceleration α is described in terms of $radian/s^2$.

Question 4: The rate at which the angular velocity changes with respect to the time is known as ______.

1. Angular velocity
2. Angular displacement
3. Angular speed
4. Angular acceleration

Answer: The correct option is (c).

Explanation: The rate of change of angular velocity is known as angular acceleration.

Question 5: One radian equals the angle of______ degree.

1. 47.3°                                                            
2. 57.3°
3. 55.6°                                                            
4. 33.2°

Answer: The correct option is (b).

Explanation:

We know that,

$2\pi \mathrm{radians} =360°$

$\pi \mathrm{radiands} =180°$

$1 \mathrm{radian} = \frac{180°}{\pi }$

$1 \mathrm{radian}=57.29°$=$57.3°$